Skip to main content

Beam Deflections - Moment - Area Method

Moment - Area Method - Mohr's Theorems 

Otto Mohr (1835-1918)
 German Civil Engineer
In this method, to determining the slopes and deflections in beams involves the Area and Moment of the 'Bending moment diagram'. 
Deviation and Slope of Beam by Area-Moment Method
( B.M.D )
First Theorem :
The angle between tangents drawn at any two points on deflected curve, is equal to area of the M/EI diagram between the two points.
The angle between the tangents from points A and B 
O AB =  1/EI (Area of AB)

Second Theorem :
The intercept on a vertical line made by two tangents drawn at the two points on the deflected curve is equal to the moment of the M/EI diagram between two points about the vertical line.
The intercepts at point A,and B are,
t A/B = Z AB  =1/EI (Area of AB). XA
  and
t B/A = Z BA  = 1/EI (Area of AB). XB
Sign Convention :
For +ve B.M. ,the area of  M/EI diagram is considered +ve , and for -ve B.M.,the area of M/EI diagram is considered as -ve  

  1. 1.Slope :
  1. Measured from left tangent, if θ is anti-clockwise, the change of slope is positive, negative if θ is clockwise.
  1. 2. Deflection
    1. The intercept  at any point is positive if it lies above the tangent, negative if the it is below the tangent.
      2.

    3. Application of Moment - Area Method for Cantilever Beams

        1.  

          General representation of deflection of cantilever beams

           

          From the figure above, the deflection at B denoted as δB is equal to the deviation of B from a tangent line through A denoted as tB/A. This is because the tangent line through A lies with the neutral axis of the beam.
          Similarly the angle between the tangents will equal to slope of the point. 
          2.

        2. Application of Moment - Area Method for Simply supported Beams


          The deflection δ at some point B of a simply supported beam can be obtained by the following steps:

          1.  

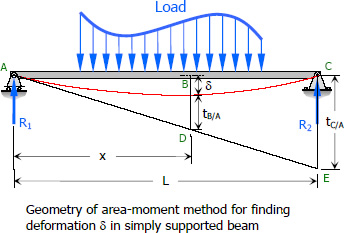          Area-moment method of finding deflections in simply supported beam


          1. Compute the vertical intercept between Cand A  = ZCA
          2. Compute the vertical intercept between B and C  = ZBA
          3. Slope at support A = O A = t CA /L = ZCA / L
          4. Slope at support C = O C = tCA/ L = ZCA / L
    4.  Generally, the tangential deviation t is not equal to the beam deflection. In cantilever beams, however, the tangent drawn to the elastic curve at the wall is horizontal and coincidence therefore with the neutral axis of the beam. The tangential deviation or vertical intercept  in this case is equal to the deflection of the beam as shown below.
Labels:

Comments

Post a Comment

Popular posts from this blog

PORTAL METHOD and CANTILEVER METHOD

The behavior of a structure subjected to horizontal forces depends on its height to width ratio. The deformation in low-rise structures, where the height is smaller than its width, is characterized predominantly by shear deformations. In high rise building, where height is several times greater than its lateral dimensions, is dominated by bending action. To analyze the structures subjected to horizontal loading we have two methods. Portal method  and Cantilever method 1. PORTAL METHOD The portal method is an approximate analysis used for analysing building frames subjected to lateral loads such as Wind loads/ seismic forces.  Since shear deformations are dominant in low rise structures, the method makes simplifying assumptions regarding horizontal shear in columns.  Each bay of a structure is treated as a portal frame, and horizontal force is distributed equally among them. Assumptions in portal method   1. The points of inflection are located at the mid-height of e...
5 comments
Read more

Support reactions in beams due to an applied couple or moment

  couple of Forces A couple is defined as two parallel forces with the same magnitude    but opposite in direction separated by a perpendicular distance d. The net external effect of a couple is that the net force equals zero and the magnitude of the net moment equals = F.d  The resultant of a couple at any point is always a pure constant moment  and equal to        M = F d Problem : 2.7 A 6 m-long simply supported beam carries a anti clockwise couple 400 N-m and a point load as shown in Fig. Calculate support reactions Solution: Let the vertical reaction at support A is Ra and at support B is Rb . Here the vertical forces are upwards reactive forces Ra ,Rb and downward point load of 1000 N. The horizontal pair of forces are formed as a couple and its resultant is a moment of 400N-m Apply the  equilibrium equation is: ∑ Fy = 0 R a  + R b  - 1000 = 0       R a  + R b    = 1000...
12 comments
Read more

Relation between Modulus of Elasticity and Modulus of Rigidity

Modulus of Elasticity (E)   It is the ratio between Normal stress to Normal strain within the elastic limit. Elastic Modulus E = Normal stress/Normal strain E =  s/e Modulus of Rigidity (G)  It is the ratio between Shear stress to Shear strain within the elastic limit. Rigidity Modulus G = Shear stress/ Shear strain G =    Ƭ / ø   Relation between Modulus of Elasticity and Modulus of Rigidity: Consider a solid cube  PQRS is  subjected to a shearing force F.  Let  Ƭ     be the shear stress produced in the faces PQ and RS due to this shear force. The complementary shear stress consequently produced in the vertical faces PS and RQ is also equal to same and shown in figure as Ƭ   Due to the pure shearing force, the cube is deformed PQRS to PQR'S' . The point   S moved to S' and point R moved to R' as shown in fig.  The shear strain = The angle of distortion  ø           ...
5 comments
Read more